All published articles of this journal are available on ScienceDirect.
Non-Resonant Frequencies of Electromagnetic Fields in α-Helices Cellular Membrane Channels
Abstract
Background:
This paper would be a starting point addressed to a methodology to minimize the effects on livings of man made Electromagnetic Fields (EMFs) pollution.
Methods:
Given that previous literature highlighted that the most relevant EMFs effects on biological systems can be due to resonance phenomena between electromagnetic field and organic matter, it was proposed here an algorithm to obtain values of frequencies of an applied electromagnetic field far from resonant frequencies, depending on the natural frequencies and viscous damper of a biological system. These frequencies have been named non-resonant frequencies.
Results:
The displacement of the α-helices in cellular membrane channels due to EMFs has been proposed as a relevant parameter for quantifying the result of the interaction between an applied EMF and organic matter, in order to find both the natural frequencies of a biological system and the resonant frequencies at which α-helices displacement should be maximum.
Conclusion:
The non-resonant frequencies can be obtained using the algorithm proposed here.
1. INTRODUCTION
The development of modern technology has induced an enormous increasing of the use of electric power devices working at electromagnetic fields frequencies at 50 or 60 Hz (named Extremely Low Frequencies Electromagnetic Fields – ELF-EMFs) and of broadcasting and mobile stations working at High Frequencies Electromagnetic Fields (HF-EMFs) up to a few GHz.
Regarding harmfulness to human health of EMFs a very large scientific production has been published by single researchers and some valuable studies have been published also by international working groups [1-6]. Some relevant health effects that have been highlighted in [1-6] are neurodegenerative and cardiovascular disorders, alterations of immune system responses and reproduction in animals and in humans, genotoxic effects, increasing of global incidence of breast cancer, childhood leukaemia and general carcinogens.
The amount of these results has induced the International Commission on Non-Ionizing Radiation Protection (ICNIRP) to publish international guidelines in order to identify exposure limits of EMFs that are recommended not to be exceeded [7-11].
Nevertheless, recent studies have showed that significant effects occur in simple organic systems even below the EMFs limits recommended by the ICNIRP [12-24]. In particular, these studies showed that significant transitions from proteins α-helix component to β-sheet structure (that represents proteins unfolding) and orientation towards the direction of the applied field occur under exposure to EMFs below the limits recommended by the ICNIRP.
Proteins unfolding is responsible for aggregation mechanisms, so that previous results lead us to hypothesize that EMFs can be a cofactor for some diseases. Indeed, proteins aggregation can be considered the precursor of various neurological disorders such as Alzheirmer, Parkinson and Huntington, because proteins aggregation in the fibrillar form (the amyloid) can be associated with signs of neurodegeneration and some forms of anemia [25-33].
Some studies showed that some bioprotectors can induce shielding action against exposure to EMFs [34-39]. Nevertheless, we do not know what may be the side effects in humans living functions of a continuous use of such bioprotectors.
However, previous studies hypothesized that natural frequencies in livings could be close to the frequencies of man-made EMFs, so that a resonance phenomenon could occur amplifying the result of the interaction mechanisms between EMFs and organic matter [40-48]. Following this scenario, this study starts from the assumption that, similar to the existence of frequencies at which a resonance phenomenon between EMFs and organic matter occurs, there may be frequency regions with an opposite effect to that of resonance, in which the effects of interaction between EMFs and organic matter are minimal. We have named such frequencies ‘non-resonant frequencies’. In order to hypothesize to plan electronic devices working at such frequencies, this study proposed an algorithm to calculate the non-resonant frequencies of EMFs as a function of natural frequencies of organic matter. In particular, the displacement of α-helix in cellular membrane channels due to the interaction with EMFs has been proposed as a parameter useful to quantify harmful effects of EMFs on livings, given that it was shown that the orientation of α-helices in cellular membrane channels along the direction of the applied field should induce an increase of ions flux across the channel, changing cellular functions [24]. The existence of α-helix structure in proteins was proposed in 1951 by Linus Pauling and its existence was confirmed by X-ray crystallography. The α-helix consists of 3.6 amino-acid residues per turn in the polypeptide chain which is stabilized by hydrogen bonding between the amide hydrogen of one peptide bond and the carbonyl oxygen of another. The α-helix structure has a fundamental role in livings because it is present in all types of biological membranes [49-51].
The aim of this study was to propose an algorithm to find the non-resonant frequencies at which α-helices displacement be minimum, in order to minimize the effects of EMFs on livings as it is explained in the next sections.
2. METHODS
2.1. The Non-Resonant Frequencies Algorithm
Resonance is a phenomenon represented by the oscillation at high amplitude of a material, which occurs when the material is subjected to an external forcing at a specific frequency known as resonant frequency. When the frequency of the external forcing is close to a natural frequency of the material, a large vibration of the system is produced giving rise to the phenomenon named resonance.
Generally, a material has more than one resonant frequency. Each substance has some natural vibration frequencies that are characteristic of the material, depending on the frequencies of free vibrations of the molecules forming the material. The fundamental frequency is the lowest natural frequency, which gives rise to the maximum-amplitude oscillation of the system if it is stressed by an external forcing at that frequency. The frequencies at which a material will vibrate with large amplitude if it is forced are the natural frequencies of the material.
In contrast, if the system will be exposed to frequencies very different from its resonant frequencies, the system would not vibrate significantly.
Obviously, also biological systems are expected to have resonance frequencies in some regions of the electromagnetic spectrum. In particular, in previous literature it was proposed that biological systems can exhibit natural resonant frequencies in the microwave region [40, 52-56].
First, in order to study the interaction between EMFs and organic matter, an appropriate model has to be chosen to represent the response of biological systems to EMFs.
However, given that a biological system is a complex system, it would be represented by a large number of natural frequencies that cannot all be taken into account. Thus, we focused on cellular membrane channels α-helices, because their displacement due to EMFs should change the delicate equilibrium of ions flux across cells membrane channels [21, 24].
The α-helix behavior in cellular membrane channels in response to an applied EMF can be described by a viscoelastic model by means of three parameters, the spring, damper and mass elements. The modeling by a viscoelastic model of a generic organic system under an applied EMF was already proposed by [57, 58].
The external force acting on a charged particle can be represented by the term qE, in which q is the charge of the organic system and E is the electric field component of the EMF, which can be expressed by a sinusoidal function:
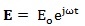 |
(1) |
Generally, this term is correlated to the magnetic component by Maxwell’s equations. Nevertheless, it was shown that the far-field approximation can be applied to the EMF emitted by wireless devices at working distance, whose the most representative is mobile phone [59]. In this case, the electric and magnetic field components are closely related as the ratio of the electric field to the magnetic field component is given by:
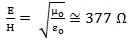 |
(2) |
where μ o and ε o are the permeability and permittivity of free space, respectively, so that it is sufficient to evaluate only one of them.
Furthermore, given that the organic system exposed to EMF should have non-negligible dimension, the torque induced by the EMF on the system has to be taken into account. In addition, it has to be compared to the Brownian motion due to thermal agitation, to which the organic system embedded in aqueous environment is subjected. To this aim, the angular impulse from EMF, that is ΔE*μ, should be compared with the mean angular momentum from thermal agitation
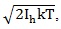 , in which Ih is the moment of inertia of the organic system. Adair performed a simulation using hemoglobin as a typical simple organic system [60], providing the result that the mean angular momentum from thermal agitation is larger than the impulse from an applied electric field with amplitude E=100 kV/m. Nevertheless, the simulation of [60] can be applied to a dipole exposed to a EMF in the vacuum. In contrast typical organic systems such as cells or proteins α-helices can be considered macrodipoles embedded in a aqueous medium whose viscosity cannot be neglected, so that they cannot follow the oscillation of the applied HF-EMF due to the inertia of molecules and the viscosity of the medium, placing theirself at an average position along the direction of the field [24]. However, recently it was shown that 3 h exposure to EMFs of hemoglobin in aqueous solution samples induces that the impulse from the magnetic field overheads the mean angular momentum from thermal agitation [61]. The term due to thermal molecular agitation represents the viscosity of the medium in which the organic system is embedded can be taken into account by the damper coefficient of the medium ν.
, in which Ih is the moment of inertia of the organic system. Adair performed a simulation using hemoglobin as a typical simple organic system [60], providing the result that the mean angular momentum from thermal agitation is larger than the impulse from an applied electric field with amplitude E=100 kV/m. Nevertheless, the simulation of [60] can be applied to a dipole exposed to a EMF in the vacuum. In contrast typical organic systems such as cells or proteins α-helices can be considered macrodipoles embedded in a aqueous medium whose viscosity cannot be neglected, so that they cannot follow the oscillation of the applied HF-EMF due to the inertia of molecules and the viscosity of the medium, placing theirself at an average position along the direction of the field [24]. However, recently it was shown that 3 h exposure to EMFs of hemoglobin in aqueous solution samples induces that the impulse from the magnetic field overheads the mean angular momentum from thermal agitation [61]. The term due to thermal molecular agitation represents the viscosity of the medium in which the organic system is embedded can be taken into account by the damper coefficient of the medium ν.
Finally, the equation of motion of this viscoelastic model which can represent the behavior of the organic system exposed to EMFs is represented by the following equation:
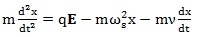 |
(3) |
in which m is the mass of the system, ν is the damper coefficient, k is the spring element of the system and ωs is a fundamental frequency of the system. In addition,
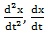 and x are, respectively, the acceleration, velocity and complex displacement of a particle which is subjected to the applied electric field.
and x are, respectively, the acceleration, velocity and complex displacement of a particle which is subjected to the applied electric field.
The generic solution of Eq. (3) is as follows:
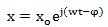 |
(4) |
where x o and φ are the amplitude of displacement and the phase angle, respectively, that are to be calculated. Let we consider that the oscillation of the system begins with beginning of exposure to the external field, so that it is φ = 0. Hence, we may rewrite the Eq. (4) as follows:
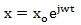 |
(5) |
The corresponding velocity and acceleration of the charged particle are, respectively,
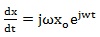 |
(6) |
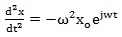 |
(7) |
Solving Eq. (3) for the displacement, putting x = x o for simplicity, we get:
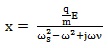 |
(8) |
A resonance phenomenon occurs when the frequency of the applied field is close to a fundamental frequency of the system ω = ωs, giving the maximum value of displacement x which is provided by Eq. (8).
Rationalizing the denominator of Eq. (8) where the imaginary unit appears, the Eq. (8) can be written as follows:
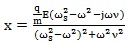 |
(9) |
The algorithm proposed here is based on the assumption that values of frequencies ω that minimize the displacement of a charged particle in Eq. (9) exist and can be found using the mathematical condition that the first derivative of Eq. (9) is equal to zero:
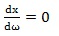 |
(10) |
This mathematical condition is expressed by the following equation:
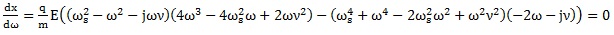 |
(11) |
Solving the Eq. (11) with respect to the frequency ω of the applied electric field we get:
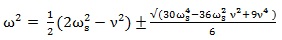 |
(12) |
The frequency values ω can be obtained by Eq. (12) as a function of the natural frequency ωs and of the damper coefficient values of an organic system. These frequency values ω should minimize the displacement x of charged particles exposed to an applied electric or magnetic field and can be named non-resonant frequencies. This procedure can be easily extended to an applied HF-EMF.
In conclusion, it was shown that given an EMF acting on an organic system, the smallest displacement of the organic charged particles exposed to the field can be found at particular frequencies of the applied EMF, that are correlated to the natural frequency and damper coefficient of an organic system.
2.2. Applying the Non-Resonant Frequencies Algorithm
Given that Eq. (12) can provide the non-resonating frequencies in any biological system as a function of natural frequency ωs and damper coefficient ν, the problem seems to be easily solved by knowing these values.
Nevertheless, the greatest difficulty is just knowing the natural frequencies ωs and damper coefficient ν in an organic system.
Previous literature provided some values of natural frequencies in some organic systems. For instance, some authors proposed the possibility that biological systems can exhibit natural frequencies in the MWs frequencies range [43-48, 52-58, 62]. [63] proposed resonance frequencies at 41.3 and 51.7 GHz for the bacterium Escherichia coli.
Nevertheless, a biological system is very complex system so that its natural frequencies cannot be easily found. In order to find these frequencies, we can choose one of the most representative parameters of a biological system whose interaction with an applied EMF gives rise to the maximum change in the livings functions of the organism.
3. RESULTS
In our opinion, this physical-chemical parameter which has a fundamental role in the resonance phenomenon with an applied EMF can be the flux of ions across cellular membranes, because its alteration changes the delicate equilibrium inside the cell altering cell viability. Indeed, ion channels in cellular membranes have been demonstrated to have a fundamental role in cellular functions [64-67].
In this regard, the displacement of α-helices in cells membranes channels caused by resonant frequencies of an EMF should alter the flux of ions across the channels, inducing damaging of cells [24]. In contrast, the application of an EMF with non-resonant frequencies should induce lesser α-helices displacements than an EMF with resonant frequencies.
In this scenario, the resonant frequencies in cellular membrane channels can be found measuring the displacement of the α-helices in membranes channels as a function of the frequency of an applied EMF, following the protocol accurately described in [13, 15]. For instance, Circular Dichroism (CD) spectroscopy can be used to measure the shift of the alpha helix with respect to its resting position in the cellular membrane channels as a function of the frequency of an applied EMF. CD is a spectroscopic technique which is generally used to study the secondary structure of proteins and in particular of membrane proteins [68]. However, this technique may be coupled with more sophisticated techniques such as X-ray crystallography or NMR spectroscopy. CD spectroscopy has the advantages of exploring a wide range of solution conditions and the rapid data collection. Other useful techniques to determine the orientation of proteins α-helices is the Nonlinear Sum Frequency Generation Vibrational (NSFGV) spectroscopy which can be coupled with linear vibrational spectroscopic techniques such as infrared spectroscopy and Raman scattering [69]. In previous studies measurements of α-helices tilt orientation have been carried out using CD spectroscopy, NMR and fluorescence spectroscopy and are reported in [70-72], respectively, showing that α-helices tilt orientation can be easily measured before and after exposure to EMFs in any type of tissue. In addition, it was already shown in [21, 24] that the orientation of cellular membrane channels α-helices along the direction of the applied EMF should cause the enlargement of cellular membrane channels, inducing the increasing of ions flux across cells channels and changing cellular functions.
Once the values of the natural frequencies at which the displacement of the α-helices in cellular membranes channels is maximum have been found, the values of the non-resonant frequencies can be obtained by Eq. (12) and using damper coefficient values such as proposed in [73-77].
CONCLUSION
Given the numerous evidence on the harmful effects of EMFs on livings, a strategy to reduce human exposure to EMFs was investigated. Considering that it has been highlighted in previous literature that a resonance phenomenon can occur whenever the frequency of the EMF is close to the natural frequency of the biological system invested by an electromagnetic radiation, an algorithm has been proposed here in order to obtain frequencies far from the natural frequencies of a biological system.
To this aim, the displacement of α-helices in cellular membrane channels has been proposed as a parameter aimed to measure the intensity of the interaction between EMF and organic matter, in order to find the biological natural frequencies that have the most important role in cellular functions of livings.
Once such natural frequencies have been found in typical organic tissues by measurements described at the end of previous section, non-resonant frequencies can be obtained using the algorithm here proposed, in order to hypothesize to plan electronic devices working at such frequencies, minimizing harmful effects of EMFs on livings.
ETHICS APPROVAL AND CONSENT TO PARTICIPATE
Not applicable.
HUMAN AND ANIMAL RIGHTS
No animals/humans were used for studies that are the basis of this research.
CONSENT FOR PUBLICATION
Not applicable.
CONFLICT OF INTEREST
The authors declare no conflict of interest, financial or otherwise.
ACKNOWLEDGEMENTS
Declared none.


